the art of problem solving amc 10

• Math Careers

Search form

• MAA Centennial
• Spotlight: Archives of American Mathematics
• MAA Officers
• MAA to the Power of New
• Council and Committees
• MAA Code of Conduct
• Policy on Conflict of Interest
• Statement about Conflict of Interest
• Recording or Broadcasting of MAA Events
• Policy for Establishing Endowments and Funds
• Avoiding Implicit Bias
• Copyright Agreement
• Principal Investigator's Manual
• Planned Giving
• The Icosahedron Society
• Our Partners
• Advertise with MAA
• Employment Opportunities
• Staff Directory
• 2022 Impact Report
• In Memoriam
• Membership Categories
• Become a Member
• Membership Renewal
• MERCER Insurance
• MAA Member Directories
• New Member Benefits
• The American Mathematical Monthly
• Mathematics Magazine
• The College Mathematics Journal
• How to Cite
• Communications in Visual Mathematics
• About Convergence
• What's in Convergence?
• Convergence Articles
• Mathematical Treasures
• Portrait Gallery
• Paul R. Halmos Photograph Collection
• Other Images
• Critics Corner
• Problems from Another Time
• Conference Calendar
• Guidelines for Convergence Authors
• Math Horizons
• Submissions to MAA Periodicals
• Guide for Referees
• Scatterplot
• Math Values
• MAA Book Series
• MAA Press (an imprint of the AMS)
• MAA Library Recommendations
• Additional Sources for Math Book Reviews
• About MAA Reviews
• Mathematical Communication
• Information for Libraries
• Author Resources
• MAA MathFest
• Proposal and Abstract Deadlines
• MAA Policies
• Invited Paper Session Proposals
• Contributed Paper Session Proposals
• Panel, Poster, Town Hall, and Workshop Proposals
• Minicourse Proposals
• MAA Section Meetings
• Virtual Programming
• Joint Mathematics Meetings
• Calendar of Events
• MathFest Programs Archive
• MathFest Abstract Archive
• Historical Speakers
• Information for School Administrators
• Information for Students and Parents
• Registration
• Getting Started with the AMC
• AMC Policies
• AMC Administration Policies
• Important AMC Dates
• Competition Locations
• Invitational Competitions
• Putnam Competition Archive
• AMC International
• Curriculum Inspirations
• Sliffe Award
• MAA K-12 Benefits
• Mailing List Requests
• Statistics & Awards
• Submit an NSF Proposal with MAA
• MAA Distinguished Lecture Series
• Common Vision
• CUPM Curriculum Guide
• Instructional Practices Guide
• Möbius MAA Placement Test Suite
• META Math Webinar May 2020
• Progress through Calculus
• Survey and Reports
• "Camp" of Mathematical Queeries
• DMEG Awardees
• National Research Experience for Undergraduates Program (NREUP)
• Neff Outreach Fund Awardees
• Tensor SUMMA Grants
• Tensor Women & Mathematics Grants
• Grantee Highlight Stories
• "Best Practices" Statements
• CoMInDS Summer Workshop 2023
• MAA Travel Grants for Project ACCCESS
• 2024 Summer Workshops
• Minority Serving Institutions Leadership Summit
• Previous Workshops
• Frequently Asked Questions
• Course Resources
• Industrial Math Case Studies
• Participating Faculty
• 2020 PIC Math Student Showcase
• Previous PIC Math Workshops on Data Science
• Dates and Locations
• Past Programs
• Leadership Team
• Support Project NExT
• Section NExT
• Section Officers Meeting History
• Preparations for Section Meetings
• Bylaws Template
• Editor Lectures Program
• MAA Section Lecturer Series
• Officer Election Support
• Section Awards
• Section Liaison Programs
• Section Visitors Program
• Expense Reimbursement
• Guidelines for Bylaw Revisions
• Guidelines for Local Arrangement Chair and/or Committee
• Guidelines for Section Webmasters
• MAA Logo Guidelines
• MAA Section Email Policy
• Section Newsletter Guidelines
• Statement on Federal Tax ID and 501(c)3 Status
• Communication Support
• Guidelines for the Section Secretary and Treasurer
• Legal & Liability Support for Section Officers
• Section Marketing Services
• Section in a Box
• Subventions and Section Finances
• Web Services
• Joining a SIGMAA
• Forming a SIGMAA
• History of SIGMAA
• SIGMAA Officer Handbook
• MAA Connect
• Meetings and Conferences for Students
• Opportunities to Present
• Information and Resources
• MAA Undergraduate Student Poster Session
• Undergraduate Research Resources
• MathFest Student Paper Sessions
• Research Experiences for Undergraduates
• Student Poster Session FAQs
• High School
• A Graduate School Primer
• Reading List
• Student Chapters
• Awards Booklets
• Carl B. Allendoerfer Awards
• Regulations Governing the Association's Award of The Chauvenet Prize
• Trevor Evans Awards
• Paul R. Halmos - Lester R. Ford Awards
• Merten M. Hasse Prize
• George Pólya Awards
• David P. Robbins Prize
• Beckenbach Book Prize
• Euler Book Prize
• Daniel Solow Author’s Award
• Henry L. Alder Award
• Deborah and Franklin Tepper Haimo Award
• Certificate of Merit
• Gung and Hu Distinguished Service
• JPBM Communications Award
• Meritorious Service
• MAA Award for Inclusivity
• T. Christine Stevens Award
• Dolciani Award Guidelines
• Morgan Prize Information
• Selden Award Eligibility and Guidelines for Nomination
• Selden Award Nomination Form
• AMS-MAA-SIAM Gerald and Judith Porter Public Lecture
• Etta Zuber Falconer
• Hedrick Lectures
• James R. C. Leitzel Lecture
• Pólya Lecturer Information
• Putnam Competition Individual and Team Winners
• D. E. Shaw Group AMC 8 Awards & Certificates
• Maryam Mirzakhani AMC 10 A Awards & Certificates
• Two Sigma AMC 10 B Awards & Certificates
• Jane Street AMC 12 A Awards & Certificates
• Akamai AMC 12 B Awards & Certificates
• High School Teachers
• MAA Social Media

You are here

Amc resources.

AMC Resources consists of material for teachers , parents and students to help with mathematical problem solving and to prepare for the MAA American Mathematics Competitions (AMC).

AMC Preparation Materials For Purchase

AMC Preparation Materials for Purchase  consists of flash drives of past AMC 8/10/12, AIME and USAMO contests as well as AMC 8 and 10/12 Math Club Packages. 

Student and Educator Resources

Amc m-powered.

With inquiry-driven instruction and projects highlighting comprehension, retention, and critical thinking, the AMC M-Powered site promotes learning by doing.  Prepare for competitions, attend virtual events, and access lesson plans. Whether you are an educator, student, parent, or a member of our greater AMC community, we invite you to connect! 

CURRICULUM INSPIRATIONS

Developed by james tanton ,  curriculum inspirations  is a collection of resources for middle and high school math teachers that demonstrate practical ways to engage students in the lively exploration of mathematics and mathematical thinking using problems from amc 8/10/12 contests — america’s longest-running and most prestigious mathematics competitions. now featured on m-powered., the art of problem solving.

The Art of Problem Solving  (AoPS) develops educational materials for the nation's top students in grades 2-12. The AoPS online community includes over 360,000 members from around the world, and the AoPS online school helps thousands of students each year develop tools needed for success in top-tier colleges and in prestigious math competitions.

Dummy View - NOT TO BE DELETED

Competitions

• Putnam Competition
• MAA History
• Policies and Procedures
• Support MAA
• Member Discount Programs
• Periodicals
• MAA Reviews
• Propose a Session
• MathFest Archive
• Curriculum Resources
• Outreach Initiatives
• Professional Development
• Communities

Connect with MAA

Mathematical Association of America P: (800) 331-1622 F: (240) 396-5647 Email: [email protected]

Copyright © 2024

• Terms of Use
• Privacy Policy
• Mobile Version

• Jan 1, 2021
• 12 min read

The 40 Most Important AMC Problems: Boost Your AMC Score with GLeaM!

Hello everybody! I've been receiving a lot of requests to write more competition-related articles, especially because the AMC is approaching pretty quickly: the exams will be hosted on February 4 and February 10, 2021. Today, I thought I'd take the opportunity to curate a set of my 40 favorite problems to serve as a resource for your AMC prep, grouped by category. I aimed this compilation at the intersection of AMC 10 and AMC 12, so they should cover both exams effectively. I carefully chose these to cover the widest range of topics possible, and they'll serve as a roadmap to figuring out what content you understand and what you might want to learn or review before the AMC. This is coming from what I have found personally successful in prepping both myself and my math team for the exams, so I hope you find it helpful!

There will be an answer key below to check all your answers! You'll have to search each problem individually on Art of Problem Solving's database for more expansive solutions; it's simply too much content to fit in this article. You are always welcome to discuss individual problems and solutions through the forum, the comment section, emailing me... or any other way you choose to reach me or our community!

Here are the links to AoPS's database:

AMC 10: https://artofproblemsolving.com/wiki/index.php/AMC_10_Problems_and_Solutions

AMC 12: https://artofproblemsolving.com/wiki/index.php/AMC_12_Problems_and_Solutions

Also, because Wix, which hosts this website, still does not allow LaTeX or other mathematical typesetting, the formatting below may be less than ideal, so I took the time to create a second version.

I created this better-formatted version here, which can also be downloaded and printed as a PDF: http://bit.ly/gleamamcproblems

This took a lot of effort, so make sure you check it out!

COMBINATORICS

This section includes Casework, Complimentary Counting, Venn Diagrams, Stars and Bars, Properties of Combinations and Permutations, Factorials, Path Counting, and Probability.

In order to not prematurely tip you off as to how to solve a problem, I won't reveal the topic for each problem, but for a challenge, see if you can match the topics to the problems for this combinatorics section and the other three sections! Feel free to email me to discuss this. (These lists are helpful to show you what you need to study for the AMC as well!)

1. 2002 AMC 10B Problem 18; 12B Problem 14: Four distinct circles are drawn in a plane . What is the maximum number of points where at least two of the circles intersect?

A) 8 B) 9 C) 10 D) 12 E) 16

2. 2002 AMC 12B Problem 10: How many different integers can be expressed as the sum of three distinct members of the set {1,4,7,10,13,16,19}?

A) 13 B) 16 C) 24 D) 30 E) 35

3. 2019 AMC 8 Problem 25: Alice has 24 apples. In how many ways can she share them with Becky and Chris so that each of the three people has at least two apples?

A) 105 B) 114 C) 190 D) 210 E) 380

Note: Though this problem is from the AMC 8, it resembles the level of a mid-AMC 10 problem.

4. 2020 AMC 10B Problem 5: How many distinguishable arrangements are there of 1 brown tile, 2 green tiles, and 3 yellow tiles in a row from left to right? (Tiles of the same color are indistinguishable.)

A) 210 B) 420 C) 630 D) 840 E) 1050

5. 2006 AMC 10A Problem 21: How many four-digit positive integers have at least one digit that is a 2 or a 3?

A) 2439 B) 4096 C) 4903 D) 4904 E) 5416

6. 2017 AMC 10B Problem 13: There are 20 students participating in an after-school program offering classes in yoga, bridge, and painting. Each student must take at least one of these three classes, but may take two or all three. There are 10 students taking yoga, 13 taking bridge, and 9 taking painting. There are 9 students taking at least two classes. How many students are taking all three classes?

A) 1 B) 2 C) 3 D) 4 E) 5

7. 2004 AMC 10A Problem 10: Coin A is flipped three times and coin B is flipped four times. What is the probability that the number of heads obtained from flipping the two fair coins is the same?

A) 29/128 B) 23/128 C) 1/4 D) 35/128 E) 1/2

8. 2004 AMC 10A Problem 16: The 5x5 grid shown contains a collection of squares with sizes from 1x1 to 5x5. How many of these squares contain the black center square?

A) 12 B) 15 C) 17 D) 19 E) 20

9. 2010 AMC 12A Problem 18: A 16-step path is to go from (-4, -4) to (4,4) with each step increasing either the x-coordinate or the y-coordinate by 1. How many such paths stay outside or on the boundary of the square -2 <= x <= 2, -2 <= y <= 2 at each step?

A) 92 B) 144 C) 1568 D) 1698 E) 12800

Note: <= is less than or equal to.

10. 2016 AMC 10A Problem 20: For some particular value of N, when (a+b+c+d+1)^N is expanded and like terms are combined, the resulting expression contains exactly 1001 terms that include all four variables a, b, c, and d, each to some positive power. What is N?

A) 9 B) 14 C) 16 D) 17 E) 19

Note: This is where algebra and combinatorics come together!

This section includes Sequences & Series, Distance = Rate*Time Problems, Numerical Reasoning, Median/Mean/Mode, Functional Equations, Polynomials, Logarithms, and Trigonometry.

1. 2010 AMC 12A Problem 5: Halfway through a 100-shot archery tournament, Chelsea leads by 50 points. For each shot, a bullseye scores 10 points, with other possible scores being 8, 4, 2, and 0 points. Chelsea always scores at least 4 points on each shot. If Chelsea's next n shots are bullseyes she will be guaranteed victory. What is the minimum value for n?

A) 38 B) 40 C) 42 D) 44 E) 46

2. 2017 AMC 10B Problem 7; 12B Problem 4: Samia set off on her bicycle to visit her friend, traveling at an average speed of 17 kilometers per hour. When she had gone half the distance to her friend's house, a tire went flat, and she walked the rest of the way at 5 kilometers per hour. In all, it took her 44 minutes to reach her friend's house. In kilometers rounded to the nearest tenth, how far did Samia walk?

A) 2.0 B) 2.2 C) 2.8 D) 3.4 E) 4.4

3. 2014 AMC 10A Problem 10; 12A Problem 9: Five positive consecutive integers starting with a have average b. What is the average of 5 consecutive integers that start with b?

A) a+3 B) a+4 C) a+5 D) a+6 E) a+7

4. 2018 AMC 10B Problem 20; 12B Problem 18: A function f is defined recursively by f(1) = f(2) = 1 and f(n) = f(n-1) - f(n-2) + n for all integers n > 2. What is f(2018)?

A) 2016 B) 2017 C) 2018 D) 2019 E) 2020

5. 2006 AMC 10A Problem 19: How many non- similar triangles have angles whose degree measures are distinct positive integers in arithmetic progression ? A) 0 B) 1 C) 59 D) 89 E) 178

Note: It's algebra in disguise!

6. 2013 AMC 12A Problem 14: The sequence log_12(162), log_12(x), log_12(y), log_12(z), log_12(1250) is an arithmetic progression. What is x?

A) 125 sqrt(3) B) 270 C) 162 sqrt(5) D) 434 E) 225 sqrt(6)

Note: "sqrt" represents square root and log_a(b) represents a logarithm with base a and argument b.

7. 2011 AMC 12B Problem 21: The arithmetic mean of two distinct positive integers x and y is a two-digit integer. The geometric mean of x and y is obtained by reversing the digits of the arithmetic mean. What is |x - y| ?

A) 24 B) 48 C) 54 D) 66 E) 70

8. 2017 AMC 10A Problem 24/12A Problem 23: For certain real numbers a, b, and c, the polynomial g(x) = x^3 + ax^2 + x + 10 has three distinct roots, and each root of g(x) is also a root of the polynomial f(x) = x^4 + x^3 + bx^2 + 100x + c. What is f(1)?

A) -9009 B) -8008 C) -7007 D) -6006 E) - 5005

9. 2007 AMC 12A Problem 17: Suppose that sin a + sin b = sqrt(5/3) and cos a + cos b = 1. What is cos(a-b)?

A) sqrt(5/3) - 1 B) 1/3 C) 1/2 D) 2/3 E) 1

10. 2009 AMC 12A Problem 25: The first two terms of a sequence are a_1 = 1 and a_2 = 1/sqrt(3). For n > 0, a_(n+2) = (a_n + a_(n+1))/(1 - a_n*a_(n+1)). What is |a_2009|?

A) 0 B) 2-sqrt(3) C) 1/sqrt(3) D) 1 E) 2 + sqrt(3)

Hint: What trig identity does this look like?

NUMBER THEORY

This section includes an emphasis on prime factorizations, as well as divisibility rules, Diophantine equations, Modular arithmetic, Fermat's Little Theorem, Simon's Favorite Factoring Trick, last digits (including Fermat's Little Theorem), and problems combining the reasoning of algebra with number theory.

I've posted a two-part series covering the most critical topics for AMC number theory, so make sure you check it out:

https://www.gleammath.com/post/number-theory-part-two

https://www.gleammath.com/post/number-theory-part-one

Let me know what other subjects you'd like to see articles on!

1. 2011 AMC 12B Problem 4: In multiplying two positive integers a and b, Ron reversed the digits of the two-digit number a. His erroneous product was 161. What is the correct value of the product a and b?

A) 116 B) 161 C) 204 D) 214 E) 224

2. 2013 AMC 12B Problem 9: What is the sum of the exponents of the prime factors of the square root of the largest perfect square that divides 12! ?

A) 5 B) 7 C) 8 D) 10 E) 12

3. 2019 AMC 10B Problem 19; 12B Problem 14: Let S be the set of all positive integer divisors of 100,000. How many numbers are the product of two distinct elements of S?

A) 98 B) 100 C) 117 D) 119 E) 121

4. 2017 AMC 10B Problem 14: An integer N is selected at random in the range 1 <= N <= 2020. What is the probability that the remainder when N^16 is divided by 5 is 1?

A) 1/5 B) 2/5 C) 3/5 D) 4/5 E) 1

5. 2013 AMC 10A Problem 19: In base 10, the number 2013 ends in the digit 3. In base 9, on the other hand, the same number is written as (2676)_9 and ends in the digit 6. For how many positive integers b does the base-b-representation of 2013 end in the digit 3?

A) 6 B) 9 C) 13 D) 16 E) 18

6. 2006 AMC 10A Problem 22; 12A Problem 14: Two farmers agree that pigs are worth 300 dollars and that goats are worth 210 dollars. When one farmer owes the other money, he pays the debt in pigs or goats, with "change" received in the form of goats or pigs as necessary. (For example, a 390 dollar debt could be paid with two pigs, with one goat received in change.) What is the amount of the smallest positive debt that can be resolved in this way?

A) 5 B) 10 C) 30 D) 90 E) 210

7. 2013 AMC 10B Problem 24: A positive integer n is nice if there is a positive integer m with exactly four positive divisors (including 1 and m) such that the sum of the four divisors is equal to n. How many numbers in the set {2010, 2011, 2012, ..., 2019} are nice?

8. 2017 AMC 10A Problem 20; 12A Problem 18: Let S(n) equal the sum of the digits of positive integer n. For example, S(1507) = 13. For a particular positive integer n, S(n) = 1274. Which of the following could be the value of S(n+1)?

A) 1 B) 3 C) 12 D) 1239 E) 1265

9. 2010 AMC 10A Problem 24; 12A Problem 23: The number obtained from the last two nonzero digits of 90! is equal to n. What is n?

A) 12 B) 32 C) 48 D) 52 E) 68

10. 2007 AMC 12B Problem 23: How many non-congruent right triangles with positive integer leg lengths have areas that are numerically equal to 3 times their perimeters?

A) 6 B)7 C)8 D) 10 E) 12

Hint: It's number theory in disguise! Look up Simon's Favorite Factoring Trick.

This section includes similarity, triangles (including various triangle area formulas), circles (including Power of a Point), quadrilaterials (including cyclic quads), the Pythagorean Theorem, angles, 3D geometry, transformations, and geometric probability. Make sure you also know the Law of Sines, the Law of Cosines, and the Angle Bisector Theorem. Though more obscure, you may also find Stewart's, Ceva's and Menelaus's Theorems helpful for AMC prep.

Here are several important triangle area formulas:

If you do not recognize some of these, feel free to ask! Here, a,b,c are the sides of the triangle with opposite angles A, B, C, and h_a is the altitude corresponding to side a. In addition, r is the radius of the inscribed circle, R is the radius of the circumscribed circle, and s is the semi-perimeter.

This source is also helpful to learn more about Power of a Point: https://brilliant.org/wiki/power-of-a-point/

Geometry is the most content-based subject for the AMC, so check out this more expansive formula sheet for competition geometry as well. This one definitely played a major role in my AMC prep: Tom Davis's Contest Geometry Handbook .

One more note: Solving lots of problems is always the best strategy to prepare for the AMC, especially for geometry, which is typically the subject the most students struggle with. I recommend you try to solve the following problems in multiple ways if possible and read the solutions on the AoPS database thoroughly ; there are some amazing applicable teaching moments in the solutions to all of these problems.

1. 2017 AMC 12B Problem 8: The ratio of the short side of a certain rectangle to the long side is equal to the ratio of the long side to the diagonal. What is the square of the ratio of the short side to the long side of this rectangle?

A) (sqrt(3)-1)/2 B) 1/2 C) (sqrt(5)-1)/2 D) sqrt(2)/2 E) (sqrt(6)-1)/2

2. 2000 AMC 12 Problem 10: The point P = (1,2,3) is reflected in the xy-plane, then its image Q is rotated by 180 degrees about the x-axis to produce R, and finally, R is translated by 5 units in the positive-y direction to produce S. What are the coordinates of S?

A) (1,7,-3) B) (-1,7,-3) C) (-1,-2,8) D) (-1,3,3) E) (1,3,3)

3. 2005 AMC 10B Problem 14: Equilateral triangle ABC has side length 2, M is the midpoint of AC, and C is the midpoint of BD. What is the area of triangle CDM?

A) sqrt(2)/2 B) 3/4 C) sqrt(3)/2 D) 1 E) sqrt(2)

4. 2001 AMC 12 Problem 15: An insect lives on the surface of a regular tetrahedron with edges of length 1. It wishes to travel on the surface of the tetrahedron from the midpoint of one edge to the midpoint of the opposite edge. What is the length of the shortest such trip? (Note: Two edges of a tetrahedron are opposite if they have no common endpoint.)

A) sqrt(3)/2 B) 1 C) sqrt(2) D) 3/2 E) 2

5. 2011 AMC 10B Problem 17: In the given circle, the diameter EB is parallel to DC, and AB is parallel to ED. The angles AEB and ABE are in the ratio 4:5. What is the degree measure of angle BCD?

A) 120 B) 125 C) 130 D) 135 E) 140

6. 2014 AMC 12A Problem 12: Two circles intersect at points A and B. The minor arcs AB measure 30 degrees on one circle and 60 degrees on the other circle. What is the ratio of the area of the larger circle to the area of the smaller circle?

A) 2 B) 1 + sqrt(3) C) 3 D) 2 + sqrt(3) E) 4

7. 2000 AMC 12 Problem 19: In triangle ABC, AB = 13, BC = 14, AC = 15. Let D denote the midpoint of BC and let E denote the intersection of BC with the bisector of angle BAC. Which of the following is closest to the area of triangle ADE?

A) 2 B) 2.5 C) 3 D) 3.5 E) 4

8. 2012 AMC 12B Problem 17: Square PQRS lies in the first quadrant. Points (3,0), (5,0), (7,0), and (13,0) lie on lines SP, RQ, PQ, and SR, respectively. What is the sum of the coordinates of the center of the square PQRS?

A) 6 B) 31/5 C) 32/5 D) 33/5 E) 34/5

9. 2008 AMC 12B Problem 21: Two circles of radius 1 are to be constructed as follows. The center of circle A is chosen uniformly and at random from the line segment joining (0,0) and (2,0). The center of circle B is chosen uniformly and at random, and independently of the first choice, from the line segment joining (0,1) to (2,1). What is the probability that circles A and B intersect?

A) (2 + sqrt(2))/4 B) (3*sqrt(3) + 2)/8 C) (2*sqrt(2) - 1)/2 D) (2 + sqrt(3))/4 E) (4*sqrt(3) - 3)/4

10. 2013 AMC 12A Problem 19: In triangle ABC, AB = 86 and AC = 97. A circle with center A and radius AB intersects BC at points B and X. Moreover, BX and CX have integer lengths. What is BC?

A) 11 B) 28 C) 33 D) 61 E) 72

It was quite an adventure compiling this article for all of you! I hope it helps you in the journey towards your AMC goals. Feel free to share this article or the PDF with any peers looking for AMC resources —and don't hesitate to direct them to join GLeaM as well ! If you find a particularly elegant, interesting, or revealing AMC problem, I'm also totally willing to add to this resource, so let me know!

Developing resources takes more work than writing more "traditional" articles, so make sure you let me know if this is something you're benefitting from, so I know if it's worth it to produce more. I also have lots of AMC 10/12 preparation tips, which I will likely publish as a follow-up article sometime in the near future.

Also, let me know if you have any other feedback or requests for articles! I have a few ideas in the works, and I'd love to hear what all of you think. Have a great rest of your winter breaks, and happy early new year!

• Competition Math
• 9th to 12th

Recent Posts

A Mathematical Zoo: A Wild Puzzle Compilation

Monkeying Around with Coconuts: An Interactive Exploration of a Century-Old Problem

The Pros and Cons of Math Tournaments: A Reflection on My Eight-Year Competition Math Career

2024 AMC 8 Problems

• 1 Problem 1
• 2 Problem 2
• 3 Problem 3
• 4 Problem 4
• 5 Problem 5
• 6 Problem 6
• 7 Problem 7
• 8 Problem 8
• 9 Problem 9
• 10 Problem 10
• 11 Problem 11
• 12 Problem 12
• 13 Problem 13
• 14 Problem 14
• 15 Problem 15
• 16 Problem 16
• 17 Problem 17
• 18 Problem 18
• 19 Problem 19
• 20 Problem 20
• 21 Problem 21
• 22 Problem 22
• 23 Problem 23
• 24 Problem 24
• 25 Problem 25
• 26 See Also

All the marbles in Maria's collection are red, green, or blue. Maria has half as many red marbles as green marbles and twice as many blue marbles as green marbles. Which of the following could be the total number of marbles in Maria's collection?

A chess king is said to attack all squares one step away from it, horizontally, vertically, or diagonally. For instance, a king on the center square of a 3 x 3 grid attacks all 8 other squares, as shown below. Suppose a white king and a black king are placed on different squares of 3 x 3 grid so that they do not attack each other. In how many ways can this be done?

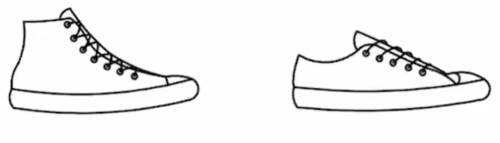
Jordan owns 15 pairs of sneakers. Three fifths of the pairs are red and the rest are white. Two thirds of the pairs are high-top and the rest are low-top. The red high-top sneakers make up a fraction of the collection. What is the least possible value of this fraction?

• AMC 8 Problems and Solutions
• Mathematics Competition Resources

Something appears to not have loaded correctly.

Click to refresh .